(This is the first part of a two part series. Part 2: 6 Ways Parents (and Teachers) Can Boost Math Learning is available here)
I hated math as a kid. But since I was good at memorizing, I was “good at math.” Then, in high school, I had to work even harder to make sense of things, and that made me hate math even more. I was definitely not “a math person.”
But all of that changed when I went to college and worked on my elementary education degree. I was required to take two foundations of math classes in addition to my methods classes. These classes provided an in-depth look at not only how the math works, but WHY.
Suddenly, I loved math. It was my new favorite subject.
But most parents don’t get the opportunity to explore math the way I did as an apprentice teacher. So when kids come home with math homework that models multiplication in different ways, old math insecurities may come back. As a parent, you may feel like you, too, are “not a math person” and want to jump to a method that’s familiar to you.
Please don’t.
If you don’t identify as a “math person,” it may mean that your early math experiences didn’t build great conceptual understanding. The so-called “new math” being taught today is trying to change that.

The Real Work of Mathematics
The ways that schools have traditionally taught math often emphasized rote procedures, repetitive practice, and speed. These are the qualities we often think of as “old math,” and no wonder it bred generations of adults with negative feelings toward math. In reality, math is creative and conceptual. Some mathematicians dedicate their entire careers toward working out a single math problem. They don’t race through a page of computations to prove how fast they are. They look for patterns, build connections, and think deeply. And that is what many of the modern approaches to teaching math encourage as well.
Jo Boaler, a professor of mathematics education at Stanford University, cites cutting-edge brain research in her book Mathematical Mindsets. She says “everyone, with the right teaching and messages, can be successful in math, and everyone can achieve at the highest levels in school.” She makes a small exception for students with very specific special education needs (no more than 5% of the population). But her brain research shows that brains can build sophisticated connections from a few minutes of practice each day over a few weeks. The idea that certain people are born “math people” is a damaging myth.

Why There’s Resistance
Over the years, I’ve heard several arguments against the “new math.” These are often associated with the Common Core State Standards that came out in 2010. Change is hard, especially for parents who never felt great about math themselves. Math anxiety is real. That’s a big burden for many of us to overcome.
Here are some of the criticisms I’ve heard and some new ways to think about it.
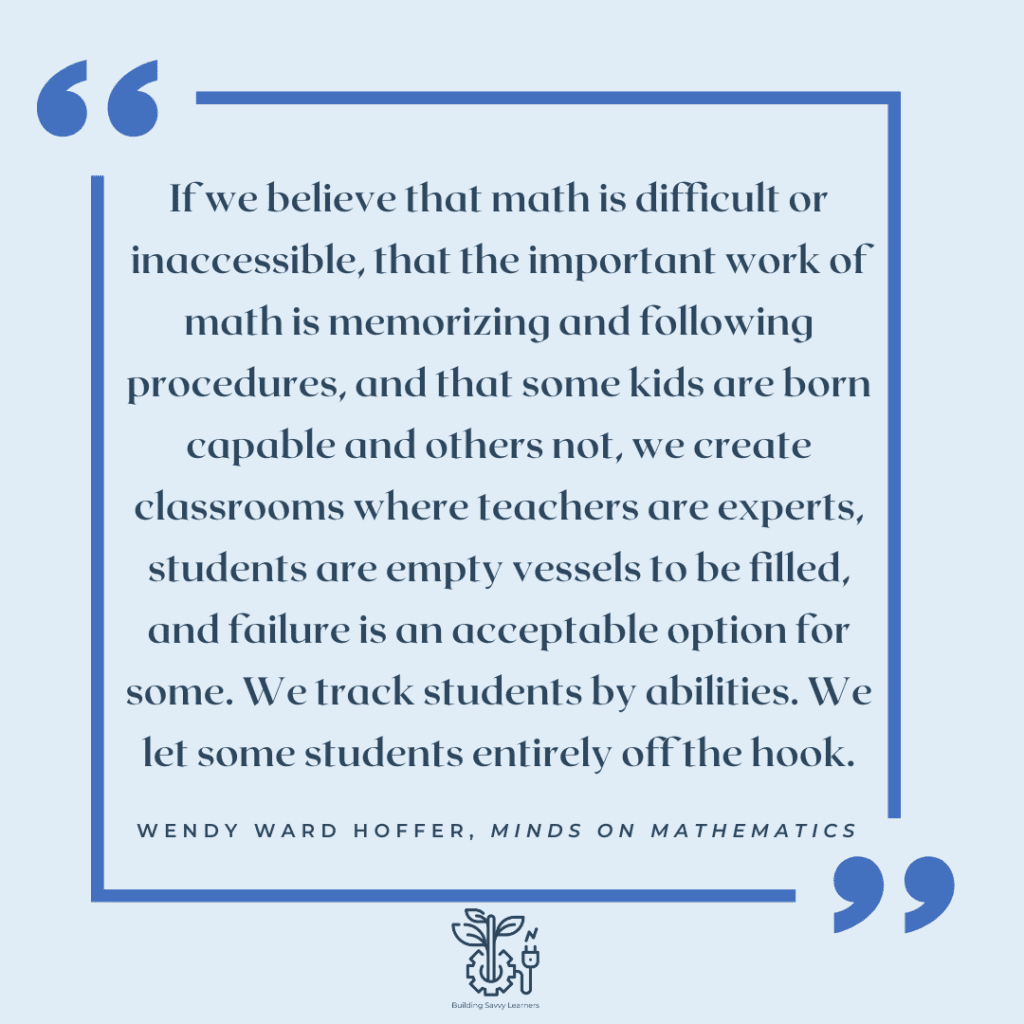
Argument: The old way worked just fine for me.
I’ll assume that you like math and don’t have any of the math anxiety I already mentioned. There are two issues that still need to be considered. First, the “old way” often valued students as “gifted” or “strong” mathematicians based on the speed and accuracy of their work. This is damaging for a lot of students—especially girls. Current research suggests that this fixed mindset discourages all students—including high-achievers—from tackling challenges because they fear being seen as a fraud (Boaler, 2016; Dweck, 2006). They worry that people won’t think they’re good at math anymore, so they become afraid to advance to the highest levels. This is part of the reason women are underrepresented in math and science fields.
Second, math is more about the process than the outcome. We want students to be critical thinkers who can reason and solve problems. We want them to notice patterns and make connections. While the ability to compute may be valuable, that’s not the end game. We have calculators for that. Learning to see math as being more than just the numbers helps students think more flexibly and creatively—skills that will benefit them in any field in the long run.

Argument: The visuals and drawings are a waste of time.
Math educators have been studying the process of how students learn math for a long time, and a lot of research supports a model called CRA: concrete – representational – abstract (see e.g., Miller & Mercer, 1993). This approach says that learners develop the best understanding of a concept when they start with physical (concrete) manipulatives like counters or base-ten blocks. Hands-on tools help kids explore an unfamiliar concept because they can move the materials around and see how a process works.
Once students have a good sense of the concrete level, they move onto the representations. These are where the visuals and drawings come into play. Students use the pictures to represent their thinking. According to Ennis and Witeck (2007), “finding ways to represent their ideas pushes students to think more deeply about those ideas as they determine ways to communicate them to others.” Also, this work with visuals activates a different part of the brain than the numbers alone. Thus, this builds stronger connections for lasting learning.
Once students know how to represent their thinking, they can move onto abstract thinking with the numbers alone. By this stage, they have a better understanding of why processes work. They can also use the concrete or representational tools to prove their solution. Jumping ahead to the abstract level without the prior work results in kids who are simply memorizing algorithms. They won’t have the deeper understanding to know how the strategy works and why. They’ll also be less flexible when introduced to new concepts because they won’t have the depth of background knowledge to build off of. The investment in visuals and drawings can buy deeper understanding in the long run.

Argument: One strategy is enough. Why confuse kids with multiple strategies?!
This is a tricky one. My answer to this depends on the goal. If the goal is to solve problems for some math test, then yes — one strategy deeply understood is probably fine. But if the goal is to have a deeper understanding of math concepts, then it’s better to look at multiple strategies and build connections between them. Here’s an example for 2 digit by 2 digit multiplication.
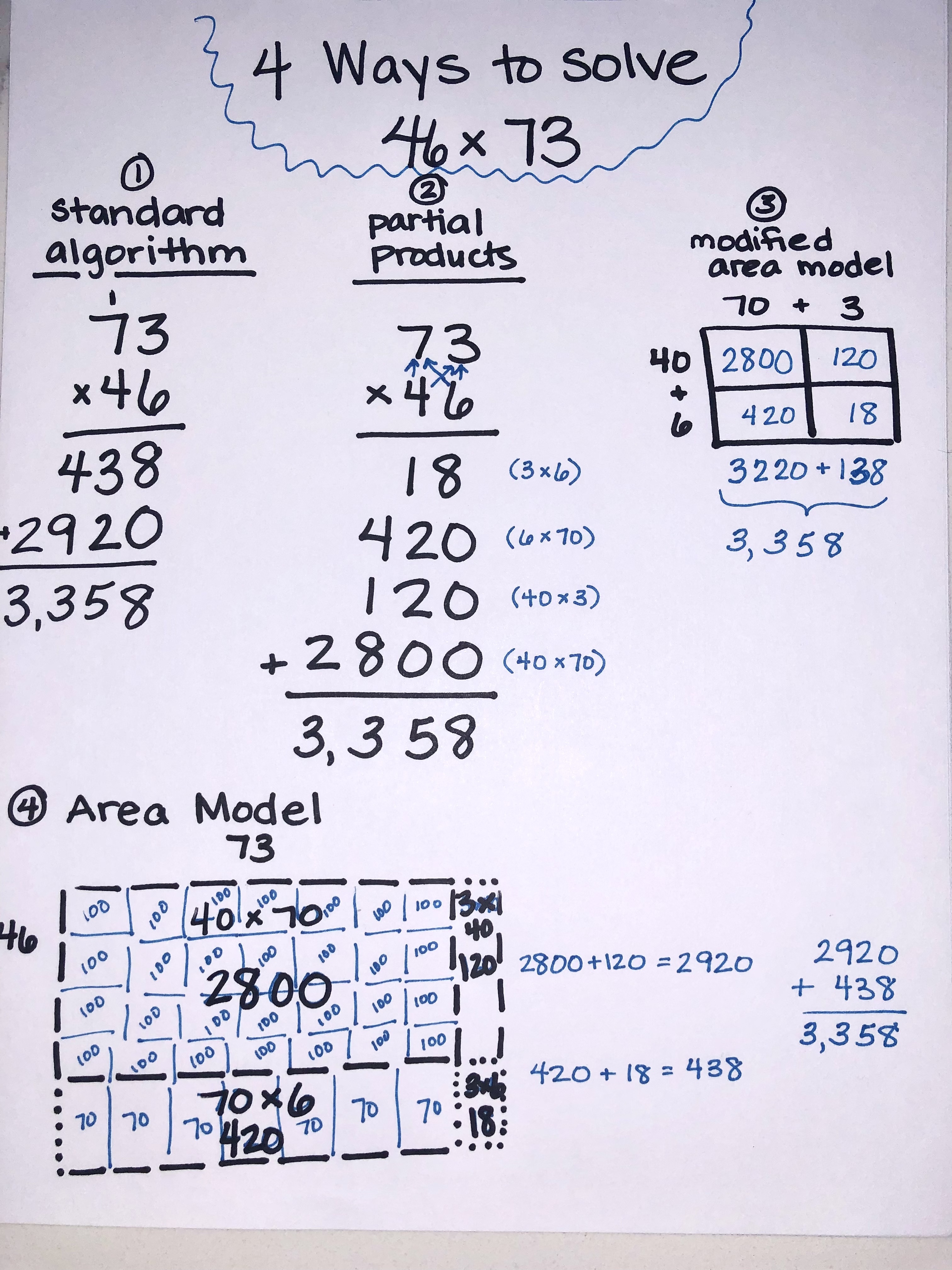
I purposefully set this up in the reverse order I would teach it so you can see some of the connections.
Process 4: The Area Model
The area model (process 4) is pretty elaborate. I would have students build these with base ten blocks first so that they could see that every time we multiply 10×10, we get 100. There are also times when we’re multiplying 1s by 10s, and 1s by 1s, so students would need the concrete and representational opportunities to see that different blocks are needed. This develops the understanding of place value.
Process 3: The Modified Area Model
From there, we can look at the modified area model (process 3) to use those place value concepts to multiply without needing to build it all out. We’re just solving four problems: 40×70, 40×3, 6×70, and 6×3 and then adding those answers together.
Process 2: Partial Products
Partial products (process 2) is the bridge between the area models and the standard algorithm. It helps students see that there are four problems to be solved. (A lot of kiddos without this understanding will only do 2 problems: 6×3 and 40×70 and get frustrated when they get the wrong answer). It also explains why you put that zero in when you move to the tens place with the 4. It’s actually 40, not 4, but anytime you multiply something in the tens place, the answer ends in a zero.
Process 1: Putting it all together in the Standard Algorithm
The standard algorithm (process 1) combines all of that understanding into an efficient strategy that hopefully kids will understand. But the other representations are what will get them to that understanding. It will also make it easier for kids to expand the pattern to include 3 digit numbers and beyond.
Plus, one size doesn’t fit all. I actually prefer the modified area model over the standard algorithm because it incorporates more mental math. Other learners like the process of partial products so they don’t skip steps. There’s not a single “right way” to solve a problem, and we need to teach kids to be flexible in their approaches.
Looking Ahead
This is an exciting time for math research. Innovations in technology like functional MRIs are helping us understand more about how the brain learns and processes math. And as that research becomes more widely known, we can anticipate more improvements in math instruction. In the meantime, it’s important that parents support this progress and their child’s math learning. I’ll be back on Thursday with specific strategies for parents and teachers to get involved.
Be sure to read Part 2 to learn more about what parents and teachers can do to support math learning.
Read More
(Note: This section contains affiliate links. That means if you click on the link and make a purchase, I will get a small commission at no additional cost to you. To learn more about why I use affiliate links, you can read my disclosure policy.)
Boaler, J. (2016). Mathematical mindsets: Unleashing students’ potential through creative math, inspiring messages and innovative teaching. San Francisco, CA: Josey-Bass.
Boaler, J. (2019). Limitless mind: Learn, lead, and live without barriers. New York, NY: HarperCollins.
Dweck, C. S. (2006). Is math a gift? Beliefs that put females at risk. In W.W.S.J. Ceci (Ed.), Why aren’t there more women in science? Top researchers debate the evidence. Washington, DC: American Psychological Association.
Ennis, B.H. & Witeck, K. S. (2007). Introduction to representation: Grades 3-5. Portsmouth, NH: Heinemann.
Hoffer, W. W. (2012). Minds on mathematics: Using math workshop to develop deep understanding in grades 4-8. Portsmouth, NH: Heinemann.
Hyde, A. (2006). Comprehending math: Adapting reading strategies to teach mathematics, K-6. Portsmouth, NH: Heinemann.